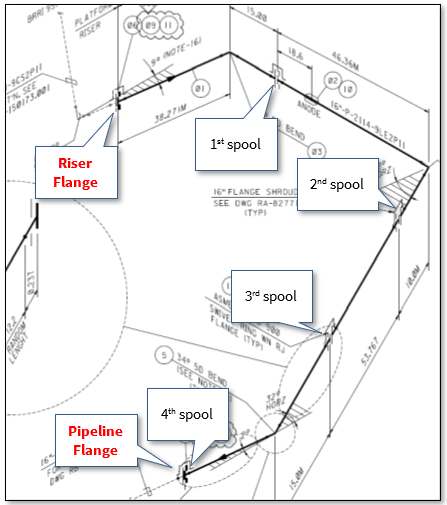
In the offshore construction industry, the connection between the newly installed pipeline and the riser is accomplished via a series of ‘spoolpieces’ (or spools). The spool is fabricated by welding pipe joints to form an L–shaped, Z–shaped, or possibly a straight pipe. The figure below shows that four spools connect the riser flange to the pipeline flange, where the first, second, and fourth spools are L-shaped, whereas the third one is a straight spool.
The lifting analysis of a subsea spoolpiece typically yields the specifications of the lifting slings and the lifting configuration, which represents the location of the pick-up (rigging) points. In addition, the analysis checks the stresses in the spools resulting from the lifting forces. However, the pipe stresses would be of concern in limited cases where we have to use a small lifting angle (with the horizontal), and the bend angle is approximately 90 degrees, coupled with a small pipe (e.g., 8 inches or less). Otherwise, lifting the spool does not usually cause stresses exceeding the allowable limits.
In all cases, we must obtain the lifting configuration; thus the tension in the wires, to correctly choose the suitable slings. That means we must find/purchase the slings matching the analysis results, which may cost much. However, the main problem is the potential delay of providing the slings if we have a tight schedule, e.g., when changing the lifting configuration of the closing tie-in spoolpiece.
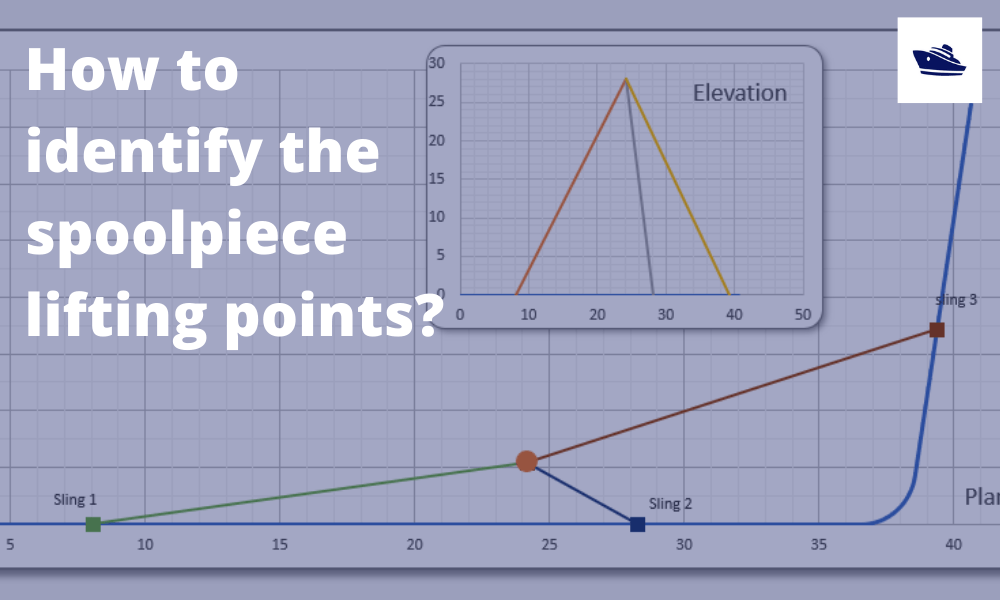
What if the lengths of the available slings become an input in the analysis procedure? In other words, the question becomes, “where can I install a sling of a particular length on the spool, with a particular hook height?” So if we were able to change the way we do the analysis, we would be using slings available in the stock, provided they have reasonable sizes and lengths, saving costs in many ways. Is that possible?
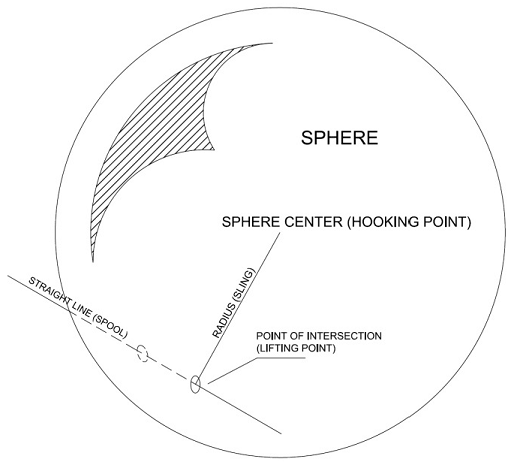
The answer is yes. The basic idea of obtaining the location of the pick-up points is that they represent the intersection between a sphere and a straight line, but how?
The sphere is centered at the hooking point, and its radius is the sling length. That means that the sphere surface represents all the possible points in space around the hooking point that are at an equal distance of the sling length from it. These points, of course, include the pick-up points we are looking for; thus, our job now is to find the intersection points between the sphere and the spool straight lines. See the below illustrating sketch.
Mathematically speaking, the sphere equation is defined by the center (hooking point) and the radius (sling length). And of course, the hooking point should be at a suitable vertical distance (hooking height) from the center of gravity, which should be calculated as well. Of course, that hook height should be reasonably shorter than the shortest sling. On the other hand, the spool equation is identified by the spool pipes lengths and bends’ angles. Consequently, the two equations must be solved simultaneously, yielding, ideally, two quadratic the root(s), representing the coordinates of the pick-up points of one particular sling length on a specific spool pipe.
Furthermore, the tension in the slings can be found by simple equilibrium equations of rigid body mechanics. So we have six equilibrium equations: three for summing forces in the three coordinate directions and three for summing moments. In that case, if we imagine that the max number of slings is six (which is practically too many), we can use as many equations as the number of slings we wish to use. Ultimately, we solve these equations simultaneously, get the tension, and subsequently choose the proper slings or probably check if the tension matches the slings we have in stock.
This procedure can be done by Excel VBA. A program has been developed (link at the bottom of this article) that performs the whole process from identifying the center of gravity, getting the pick-up points, and obtaining the tension. And, of course, the user can redistribute the slings and get new tension values if he wishes. Obtaining positive tension values is a sign that the slings distribution is physically possible and stable.
The following video explains the concept graphically.

Mohamed Hermas
Founder, Offshore Engineering Guide
Mohamed is an Offshore Pipeline Engineer and a CAE specialist, holding an M.Sc. in Subsea Engineering from the University of Aberdeen. He has been working in the offshore construction industry, with a focus on pipelines and cables, for 19 years.
Hermas has served in several capacities over the course of his career. He began as a field engineer, then project engineer, installation engineer, and pipeline engineer in many places worldwide.
In 2016, Mohamed was awarded the Chartered Engineer title from the Institution of Mechanical Engineers. In the same year, he launched a website for offshore construction professionals, with the following address: offshoreengineerguide.com
Mohamed’s interest in studying the finite element method began in 2017. By 2018, he has built his first mathematical model for stress analysis by Matlab language. Subsequently, he developed solvers serving many engineering purposes using Matlab, Wolfram language, and Excel VBA. He helps companies and individuals to make tailored programs/calculators for different engineering applications for which there is no software package available in the market.
Disclaimer:
The views, information, or opinions expressed in this article are solely those of the author and do not necessarily represent those of TheNavalArch Pte Ltd and its employees

Designing the lashings of deck cargo using IMO CSS
Introduction More than 70% of the earth is covered by water, which makes shipping historically the easiest and cheapest way of connecting manufactures and customers across the globe and can be reasonably considered to be the artery of the global economy....

Using MS Excel to evaluate the Stability of existing Barges
Barges are the simplest, and yet most widely used of marine vehicles. They are used for a variety of purposes ranging from carrying cargo in bulk or liquid, to even carrying passengers for short inland cruises. Barges are mostly towed by another barge called a tug,...

The importance of ULS (Ultimate Longitudinal Strength) and how to assess it for a damaged hull
by Alessandro La Ferlita, Naval Architect Ultimate hull girder strength represents the maximum capacity, of the hull girder beyond the structure fails. In fact, if the vertical bending moment applied overcomes a certain maximum value, the ship can collapse (Figure 1)...

Designing the berth mooring of your vessel with this simple yet effective method
A vessel at berth experiences much lower forces compared to a vessel in the open sea due to the milder environment, but it still requires a mooring configuration suited to the forces it experiences, and also suitable for the type of berthing configuration adopted. The...

Understanding how buoys affect the catenary of a mooring line
What is a mooring line? Mooring lines generally comprise ropes, wires, chains or combination of wire and chain used to keep ships, offshore platforms and other floating vessels in position. It connects the structure either to the seabed using an anchor or the quay...

Calculating forces on a ship’s deck cargo – a simplified approach
A cylindrical deck cargo (Source: Wikimedia) Introduction A ship’s deck is used to transport many different types of cargo – from containers to large structures like cranes or heavy modules of an offshore production plant. During transport, the ship suffers from...

Designing a pad-eye: little items with big intricacies
Pad-eyes are one of the smallest and most universally used structural items in the maritime and Oil & Gas industry. They are used for a variety of purposes too: from a simple seafastening of a cargo to deck of a vessel, to complicated lifting operations involving...
Vessel with Stern on Quay: A simplified method for mooring design
A vessel at berth experiences much lower forces compared to a vessel in the open sea due to the milder environment, but it still requires a mooring configuration suitable to the forces it experiences, and also suitable for the type of berthing configuration adopted....

A simple method of selecting the right anchor for mooring a tanker/gas carrier
Anchoring is a fundamental and sensitive operation for a vessel. When a vessel is at anchor, it swings to align itself along the direction of the dominant environment. The anchor is supposed to hold the vessel in varying environmental conditions depending on where the...
Designing a simple 4-point lashing system for a Deck Cargo
Introduction Lashing of a deck cargo on a ship involves different means and mechanisms to secure the cargo to the deck of the ship. This ‘securing’ is important to contain the movement of the cargo in view of the ship motions during the transportation. The simplest...
Please check out TheNavalArch’s product for planing vessel resistance estimation:

Interesting solution to a practical problem.